Journal Covers
In this section, we are presenting journal covers with images resulting from research related to the BZ reaction.
We are continuously populating this section but if you know about a missing cover, please let us know!
......................................................................
This is the cover page of Science, Volume 175, Issue 4022, 11 February 1972.
The cover features an image from the article "Spiral Waves of Chemical Activity" on page 634 by Arthur Winfree.
Involute spiral waves propagating after disruption of spontaneously formed circular waves in a layer (1 millimeter in depth) of Zhabontisky-Zaikin reagent. Small circles are carbon dioxide bubbles. Rotation period is 60 seconds; propagation velocity is 3.3 millimeters per minute at 6° (actual size of dish, 9 centimeters in diameter). See page 634. [Ken Harvey, Photographic Section, MRC Laboratory of Molecular Biology, Cambridge, England]
This is the cover page of Chemie in unserer Zeit, Volume 7, Issue 6, December 1973.
The cover features an image from the article "Das Experiment: Eine oszillierende Reaktion" on page 171 by Richard Field.
Normalerweise laufen chemische Reaktionen vom Start bis zu einem Gleichgewichtszustand ab. In seltenen Fällen treten ,,Oszillationen" bei den Konzentrationen der Reaktionspartner auf, die auf unserem Titelbild als Reaktionswellen erscheinen. Die Zusammenhänge werden in dem Beitrag ,,Eine oszillierende Reaktion" auf S. 171 erläutert.
This is the cover image of Scientific American, Vol.230, Number 6, June 1974.
The cover features images of the article "Rotating Chemical Reactions" on page 82 by AethurT. Winfree
Abstract:
The photograph on the cover shows the surface of a shallow liquid in which a sequence of chemical reactions is proceeding in an array of spiral patterns (see "Rotating Chemical Reactions," by Arthur T. Winfree, page 82). The liquid, which has been named the Z reagent, is a mixture of bromine compounds, malonic acid and the indicator dye iron phenanthroline. The Z reagent is mostly red; blue waves of chemical activity propagate through it at a rate of several millimeters per minute, forming spirals or rings. Where two of the blue waves meet they annihilate each other. The small black circles are bubbles of carbon dioxide, a product of the reaction.
This is the cover page of Nature, Volume 304, Issue 5927, 23 August 1983.
The cover features an image from the article "Three-dimensional chemical waves in the Belousov–Zhabotinskii reaction " on page 611 by Brian J. Welsh, Jagannathan Gomatam, and Arthur E. Burgess.
Abstract:
A simply-connected region of the Belousov–Zhabotinskii (B–Z) reaction medium may produce a variety of three-dimensional waveforms of unusual geometry. The reaction-diffusion hypothesis even in its linear formulation supports iso-concentration surfaces which include helical surfaces and toroidal scroll waves with twists. A variety of other waveforms has been proposed by Winfree and Strogatz. Experimental difficulties associated with observations in three dimensions have precluded confirmation of these waves in situ. We now present direct experimental evidence of evolving three-dimensional chemical waves. Our design is easy to implement, lends itself to time-delay photography and is essentially free of difficulties pointed out earlier. The dominant waveforms are toroidal scrolls; other waveforms observed include the sphere-like structure predicted recently.
This is the cover page of Science, Volume 230: Issue 4726, 08 November 1985.
The cover features an image from the article "The Structure of the Core of the Spiral Wave in the Belousov-Zhabotinskii Reaction" on page 661 by Stefan C. Müller, Theo Plesser, and Benno Hess.
Abstract:
The quantitative structure of the core of the spiral-shaped traveling wave of chemical activity appearing in a thin excitable layer of the Belousov-Zhabotinskii reaction, in which the oxidation and decarboxylation of malonic acid by bromate ions is catalyzed by ferroin, was analyzed experimentally. Light absorption by ferroin as the reduced reaction catalyst and indicator was measured by means of a video-and computer-based two-dimensional spectrophotometer with 10-micrometer spatial, 2-second temporal, and 256-digital units intensity resolution. The spiral core is a singular site (diameter, 30 micrometers or less) at which intensity modulations due to ferroin-ferriin distributions are at least ten times smaller than in the surrounding area of spiral propagation. Archimedean spirals were fitted to iso-concentration lines.
Note: The journal credits only Stefan Müller for the image which Benno Hess was furious about and mentioned it to him even before S.C.M saw the actual article.
This is the cover page of Naturwissenschaftliche Rundschau,Volume 39, Issue 9, 1986.
The cover image, called "Gebändigtes Chaos (Tamed chaos)", was provided by Mario Markus, Stefan C. Müller, Theo Plesser, and Benno Hess.
The image is figure C2 from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
About the cover:
Die Aufnahne zeigt chemische Wellen in einer dünnen Reaktionsschicht, in der die Belousov-Zhabotinsky-Reaktion abläuft. Bei der Reaktion handelt es sich um den oszillierenden oxidativen Abbau von Malonsäure durch Bromat in Gegenwart katalytischer Mengen von Ferroin. Die Wellen treten in einer abgedeckten Petrischale als konzentrische Kreise oder als Spiralen auf. Nach Entfernen des Schalendeckels entstehen chaotische Muster aufgrund der Wechselwirkung der chemischen Wellen mit Konvektionsströmen, die sich durch Verdunstung ausbilden. Durch erneutes Zudecken der Petrischale organisiert sich das System wieder spontan, und es entstehen Wellenmuster, wie sie hier gezeigt werden. Das Bilad wurde mit einer Fernsehkamera aufgenommen und anschließend in einem Computer übertragenund verarbeitet. Die Linien auf dem Bild sind Kurven gleicher Konzentrationen des Indikators Ferroin. Hohe, mittlere und niedrige Ferroinkonzentrationen sind durch die Farben rotbraun, gelb und grün dargestellt. Ähnliche Wellen sind in biologischen Systemen beobachtet worden: in Bakterienkolonien, beim Schleimpilz während seiner Aggregation von einzelligen zum mehrzelligen Stadium, im unregelmäßig schlagenden (flimmernden) Herzmuskel, in der Netzhaut von Hühnern und in der Hirnrinde von Ratten und Kaninchen.
This is the cover page of Naturwissenschaften, Volume 73, Issue 4, 1986.
The cover features an image from the article "Two-Dimensional Spectrophotometry and Pseudo-Color Representation of Chemical Reaction Patterns" on page 165 by Stefan C. Müller, Theo Plesser, and Benno Hess.
The image is figure C3 (The rotating chemical spiral) from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
Abstract:
Complexity in space and time is frequently observed in the dynamical evolution of chemical reactions kept far from thermodynamic equilibrium. First observations were made more than 100 years ago, but systematic investigations on such phenomena both in theory and experiment have been carried out only during about the last two decades. The most striking features are chemical oscillations and the formation of remarkably regular spatial patterns (for recent reviews see [1–3]). Historically, the first periodic phenomena were found in electrochemical reactions [4], while liquid phase oscillations, as already reported by Bray in 1921 [5], remained unnoticed by the scientific community for over 40 years. The discovery of the Belousov-Zhabotinskii (BZ) reaction [6,7] and simultaneous observations in the area of biochemical oscillations [8] finally led, in the mid-sixties, to an appreciation of oscillatory phenomena which so far did not fit into the framework of classical thermodynamics.
This is the cover page of the Chemical & Engineering News, Volume 65, Number 13, 30 March 1987.
The cover features an image from the article "Patterns in Time and Space - Generated by Chemistry" on page 24 by Irving R. Epstein.
Abstract:
Anyone who has ever stared at waves coming into a beach or at a child soaring on a swing is familiar with the fascination of watching periodic phenomena. In contrast to their counterparts in physics, with their pendula, and in biology, with their circadian rhythms, chemists until recently have been largely deprived of such pleasures. Now, however, a growing company of researchers in chemical dynamics is becoming acquainted with the enchantments and frustrations of studying periodic and not-quite-periodic behavior. The study of chemical oscillations in time and space has evolved from a somewhat suspect, peripheral area of inquiry to a major field of chemical research with applications to fields from biology to meteorology.
Although there were indications as early as 1828 (in studies of the dissolution of metals in strong acids) that the rates of certain reactions might increase and decrease repeatedly, it was not until 1921 that William C. Bray at the University of ...
This is the cover page of Praxis Computer - Moderne Technologien für die Medizin, Volume 4, 3. November 1988.
The cover features an image from the article "????" on page ??.
The image is figure C11 (Coarse grained spiral ) from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
This is the cover page of Spektrum der Wissenschaft, Computer Kurzweil 2 in 1988.
The image is figure C6 (Attraction into the chemical core) from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
This is the cover page of Science, Volume 240, Issue 4851, 22 April 1988.
The cover features an image from the article "Chemical Waves" on page 460 by John Ross, Stefan C. Müller, and Christian Vidal.
The image is figure C8 (The Belousov–Zhabotinskii Cone) from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
About the Cover:
The singular properties of the core region and the rotation center of the spiral wave in the Belousov-Zhabotinsky reaction are shown in three-dimensional perspective. The image was obtained by an overlay of a large number of single snapshots of spiral rotation covering three full revolutions. It presents the upper envelope of the concentration variation, that is the maximum level of oxidation of the catalyst ferroin reached at each point in space. The envelope is shown in arbitrary pseudo colors. See page 460.
This is the cover page for Nature, Volume 336, Issue 6200, 15 December 1988.
The cover features an image from the article "Chemical vortex dynamics in three-dimensional excitable media" on page 662 by Wolfgang Jahnke, Chris Henze, and Arthur T. Winfree.
About the Cover:
A representation of the numerical solution of the reaction-diffusion equations; blue wavefronts surround a closed-ring vortex filament in a Belousov-Zhabotinsky reagent. Observation of the behaviour of such filaments sheds light on vortex dynamics in excitable media.
This is the cover page of Spektrum der Wissenschaft, Sonderheft 8 in 1989. The cover features an image from the article "Wellen aus der Computer-Retorte" on page 38.
The image is figure C6 from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
About the cover :
Als Spiralwellen breiten sich bestimmten chemischen Reaktionen Konzentrationsänderungen von Reaktionspartnern um ein Zentrum aus. Stefan C. Müller, Theo Plesser und Benno Hess vom Max-Planck-Institut für Ernährungsphysiologie in Dortmund haben dies mit Computerhilfe sichtbar gemacht. Das Bild beruht auf Daten eines zweidimensionalen Spekttrometers, das mit einer Videokamera die Intensität eines durch die chemische Lösung hindurchgetretenen Lichtfeldes gemessen hat. Aus der Lichtintensität wurde dann die Konzentration bestimmter Substanzen berechnet. In der farblichen Umsetzung ergibt die Konzentrationsverteilung ein Muster von hohem visuellem Reiz. Zugleich wird eine wichtige Eigenschaft chemischer Spiralstrukturen augenfällig: ein tiefes, dauerhaftes Konzentrationsloch im Zentrum. Wie sich chemische Wellen auf Heimcomputern simulieren lassen, beschreibt der Beitrag "Wellen aus der Computer-Retorte" auf Seite 38 dieses Heftes.
This is the cover page of the Journal of Chemical Education, Volume 66, Issue 3, March 1989.
The cover features an image from the articles: "Chemical generation and visualization of hydrodynamic instability: An extremely simple demonstration of self-organization" by Peter Bowers & Leonard Soltzberg. Additional relevant articles include: "A simple explanation of the salt water oscillator" by Richard M. Noyes, "Self-organization in chemistry: The larger contex" by Leonard J. Soltzberg, "The language of dynamics" by Richard J. Field, "Some models of chemical oscillators" by Richard M. Noyes, "The role of flow systems in far-from-equilibrium dynamics" by Irving R. Epstein, "Oscillating chemical reactions and nonlinear dynamics" by Richard J. Field and F. W. Schneider, "Amusement with a salt-water oscillator" by Kenichi Yoshikawa, Satoshi Nakata, Masahiro Yamanaka and Takeshi Waki, and "A simple explanation of the salt water oscillator" by Richard M. Noyes.
The four steps in pattern development in a convective system on this month's cover (described in detail on page 210) represent one of the many examples of self-organizing chemical phenomena discussed in a series of papers beginning with the State-of-the-Art Symposium on page 189 and continuing through page 212.
This is the cover page of Nature, Volume 339, Issue 6226, 22 June 1989.
The cover features an image from the article "Chemical waves on spherical surfaces" by Jerzy Maselko and Kenneth Showalter.
Abstract:
THE concentric-circular and spiral patterns exhibited by the Belousov–Zhabotinsky (BZ) reaction in thin films of solution are representative of spatiotemporal behaviour in a two-dimensional, planar excitable medium1–6. Here we report BZ chemical waves propagating on the two-dimensional surface of a sphere. A wave on the surface of a single cation-exchange bead, loaded with ferroin and bathed in BZ reaction mixture containing no catalyst, develops to form a rotating spiral. Unlike spiral waves in thin films of solution, which typically wind out to connect with a twin rotating in the opposite direction, these waves rotate from pole to pole in a single direction. The spiral winds outward from a meandering source at one pole, crosses the equator, and undergoes self-annihilation as it winds into itself at the other pole. This behaviour, which is not possible in a two-dimensional planar configuration, arises from qualitative (negative to positive) and quantitative changes in wavefront curvature as the wave traverses the spherical surface. These observations of a single spiral wave contrast with theoretical predictions7,8 of counter-rotating spirals in this geometry.
This is the cover page of Nature, Volume 347, Issue 6288, 6 September 1990.
The cover features an image from the article "Isotropic cellular automaton for modelling excitable media " on page 56 Mario Markus and Benno Hess .
Abstract:
Excitable media, exemplified by the chemical system of the Belousov–Zhabotinsky (BZ) reaction, are often modelled theoretically through the integration of sets of partial differential equations that describe their dynamics. An alternative approach is to use cellular automata1–4, which sacrifice insight into the detailed physical mechanisms for the benefit of being able to reproduce the observed patterns of behaviour at low computational cost. But the cellular automata used in previous work have been anisotropic (mainly square or hexagonal), leading to the problem that this anisotropy tends to be propagated into the patterns produced. Here we describe a means of generating isotropic cellular automata, which are able to reproduce a wide range of observed modes of behaviour ranging from spiral-type BZ patterns to structures reminiscent of turbulence.
This is the cover page of the Chemistry & Industry, Volume 5, 4 March 1991.
The cover features an image from the article "Chemical Chaos" on page 157 by Irving Epstein.
This is the cover page of MPG Spiegel, Volume 5, 19 November 1992.
The cover features an image from the article "???" on page ?? by Stefan C. Müller.
This is the cover page of Chemistry in Britain, Volume 31, 1995
The cover features an image from the article "Bringing Out the Order in Chaos" on page ?? by Kenneth Showalter.
This is the cover page of the Journal of Chemical Education, Volume 73, Issue 9, September 1996.
The cover shows "A close-up picture of the Belousov-Zhabotinsky (B-Z) reaction'', as featured in two articles, from a video sequence on the ChemDemos II videodisc (http://pubs.acs.org/doi/10.1021/ed073p874).
The B-Z reaction is also featured in the ChemDemo videodisc but also in the article "The BZ Reaction: Experimental and Model Studies in the Physical Chemistry Laboratory" by Omar Benini, Rinaldo Cervellati and Pasquale Fetto (http://pubs.acs.org/doi/abs/10.1021/ed073p865). They have taken this popular demonstration and transformed it into a laboratory experience that involves students in chemical kinetics and numerical integration for data analysis. In addition, Peter Strizhak and Michael Menzinger develop the principles that underlie the B-Z and other oscillating reactions in "Nonlinear Dynamics of the BZ Reaction: A Simple Experiment that Illustrates Limit Cycles, Chaos, Bifurcations, and Noise" (http://pubs.acs.org/doi/abs/10.1021/ed073p868).
This is the cover page of Genes & Development, Volume 10, Issue 18, 15 September 1996.
The cover features an image for the article "Propagation of traveling waves in excitable media" on page 2237 by Brian M. Sager.
About the Cover:
Shown are spiraling Belousov-Zhabotinsky (BZ) waves in a ferroin-catalyzed BZ reaction (Photograph courtesy of Stefan Müller).
This is the cover page of Physikalische Blätter, Volume 54, Issue 6, June 1998.
The cover features an image from the article "Strukturbildung in dissipativen kontinuierlichen Systemen" on page 513 by Stefan Müller and Jürgen Parisi.
Abstract:
Rollen oder hexagonale Zellen, die sich in einer dünnen, waagrechten und von unten beheizten Flüssigkeitsschicht ausbilden, sind das wohl prominenteste Beispiel für raum‐zeitliche Strukturbildung in dissipativen kontinuierlichen Systemen. In der Hydrodynamik, der physikalischen Chemie und der Halbleiterphysik gibt es zahlreiche weitere Beispiele, deren Untersuchung im Rahmen eines Schwerpunkts der Deutschen Forschungsgemeinschaft gefördert wird. Die exemplarisch herausgegriffene wellenförmige Ausbreitung von Erregungszuständen – das sind spiralförmig rotierende Strukturen in physikalischen, chemischen und biologischen Systemen – verdeutlicht die wesentlichen Merkmale dieses Forschungsgebiets und seinen interdisziplinären Charakter.
This is the cover page of Einblicke - Forschungsmagazin der Universität Oldenburg, Volume 5, 29 April 1999.
The cover features an image from the article "???" on page ?? by Jürgen Parisi and Stefan Müller.
The image is figure C7 (Spiral cores) from the exhibition "Dynamic Pattern Formation in Chemistry and Mathematics".
This is the cover page for Science, Volume 296, Issue 5575, 14 June 2002.
The cover features an image from the article "Design and Control of Wave Propagation Patterns in Excitable Media" on page 2009 by Tatsunari Sakurai, Eugene Mihaliuk, Florin Chirila, and Kenneth Showalter.
Intricate patterns of wave propagation are exhibited in a chemical reaction-diffusion system with spatiotemporal feedback. Wave behavior is controlled by feedback-regulated excitability gradients that guide propagation in specified directions. Waves interacting with boundaries and with other waves are observed when interaction terms are incorporated into the control algorithm. Spatiotemporal feedback offers wide flexibility for designing and controlling wave behavior in excitable media.
This is the cover page of Dalton Transactions: An International Journal of Inorganic Chemistry, Volume 7, 7 April 2003.
The cover features an image from the article "Dalton Perspective: Nonlinear Chemical Dynamics" on page 1201 by Francesc Sagués and Irving Epstein.
The interdisciplinary field of nonlinear chemical dynamics has grown significantly in breadth and depth over the past three decades. Its subject matter and applications encompass all branches of chemistry as well as areas of mathematics, physics, biology and engineering. In this Perspective, we present an overview of some of the key results of nonlinear chemical dynamics, with emphasis on those areas most likely to be of interest to inorganic chemists. We discuss the range of phenomenology from chemical oscillation to chaos to waves and pattern formation, as well as experimental methods, mechanistic considerations, theoretical techniques, and the results of coupling and external forcing.
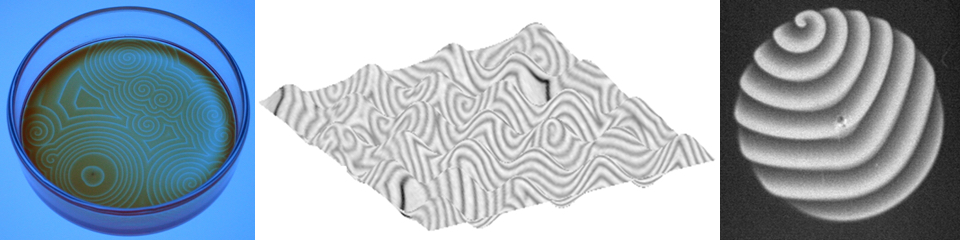
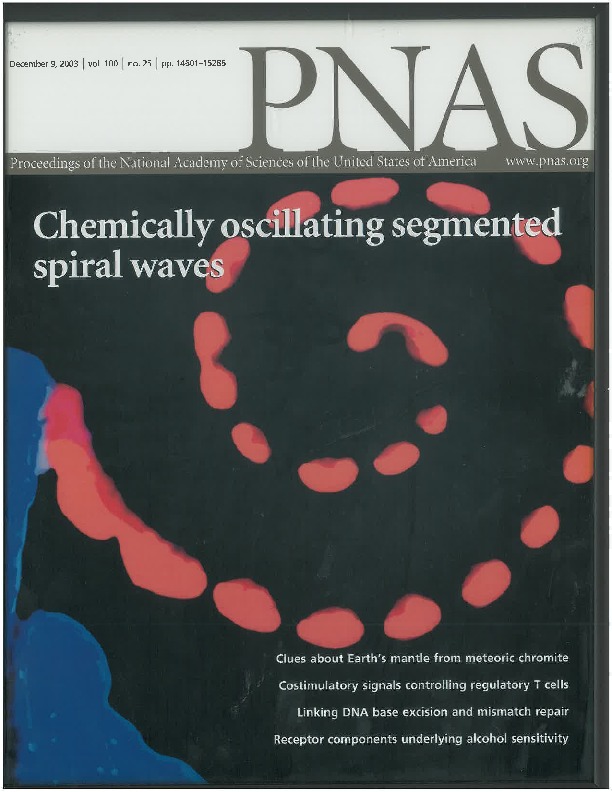
This is the cover image of Proc. Natl. Acad. Sci. U.S.A., Volume 100, Issue 25, 9 December 2003.
The cover features an image from the article "Segmented spiral waves in a reaction-diffusion system" on page 14635 by Vladimir Vanag and Irving Epstein.
Cover photograph:
Segmented spirals created by the chemically oscillating Belousov-Zhabotinsky reaction. The orange segmented spiral collides with the blue front of a striped traveling wave. Although waves annihilate when they meet, the segmented spiral continues to spawn new loops as it rotates and will eventually take over the entire frame. See the article by Vanag and Epstein on pages 14635–14638.
This is the cover page of the Journal of Physical Chemistry A, Volume 108, Issue 8, 26 February 2004.
The cover features an image from the article "Soft lithography [which] allows the study of self-organizing patterns such as spiral waves in complex microreactors" on page 1325 by Brent Ginn, Bettina Steinbock, Murat Kahveci, and Oliver Steinbock.
This is the cover image of Physical Review Letters, Volume 96, Issue 1, 4 January 2006.
The cover features an image from the article "Front Reversals, Wave Traps, and Twisted Spirals in Periodically Forced Oscillatory Media" on page 018302 by Oliver Rudzick and Alexander S. Mikhailov.
Reaction-diffusion equations are used to predict twisted spiral waves—the inner and outer parts are wound in opposite directions. The spiral retains its shape as it rotates.
Abstract:
A new kind of nonlinear nonequilibrium patterns—twisted spiral waves—is predicted for periodically forced oscillatory reaction-diffusion media. We show, furthermore, that, in such media, spatial regions with modified local properties may act as traps where propagating waves can be stored and released in a controlled way. Underlying both phenomena is the effect of the wavelength-dependent propagation reversal of traveling phase fronts, always possible when homogeneous oscillations are modulationally stable without forcing. The analysis is performed using as a model the complex Ginzburg-Landau equation, applicable for reaction-diffusion systems in the vicinity of a supercritical Hopf bifurcation.
This is the cover page of Physical Chemistry Chemical Physics, Volume 8, Issue 40, 28 October, 2006.
The cover features an image from the article 'Jumping solitary waves in an autonomous reaction–diffusion system with subcritical wave instability' on page 4647 by Lingfa Yang, Anatol Zhabotinsky, and Irving Epstein.
Abstract:
We describe a new type of solitary waves, which propagate in such a manner that the pulse periodically disappears from its original position and reemerges at a fixed distance. We find such jumping waves as solutions to a reaction–diffusion system with a subcritical short-wavelength instability. We demonstrate closely related solitary wave solutions in the quintic complex Ginzburg–Landau equation. We study the characteristics of and interactions between these solitary waves and the dynamics of related wave trains and standing waves.
This is the cover page of The Journal of Physical Chemistry A, Volume 111, Issue 13, 5 April 2007.
The cover features an image from the article "Scroll wave laments terminate in the back of traveling fronts" on page 2492 by Tamás Bánsági, Christiane Palczewski, and Oliver Steinbock.
This is the cover page of Chaos: An Interdisciplinary Journal of Nonlinear Science, Volume 18, Issue 2, June 2008.
The cover features an image from the article "Three-dimensional spiral waves in an excitable reaction system: Initiation and dynamics of scroll rings and scroll ring pairs" on page 026102 by .
Abstract:
We report experimental results on spiral and scroll waves in the 1,4-cyclohexanedione Belousov–Zhabotinsky reaction. The propagating concentration waves are detected by two-dimensional photometry and optical tomography. Wave pulses can disappear in front-to-front and front-to-back collisions. This anomaly causes the nucleation of vortices from collisions of three nonrotating waves. In three-dimensional systems, these vortices are scroll rings that rotate around initially circular filaments. Depending on reactant concentrations, the filaments shrink or expand indicating positive and negative filament tensions, respectively. Shrinkage results in vortex annihilation. Expansion is accompanied by filament buckling and bending, which is interpreted as developing Winfree turbulence. We also describe the initiation of scroll ring pairs in four-wave collisions. The two filaments are stacked on top of each other and their motion suggests filament repulsion.
This is the cover page of Journal of Chemical Physics, Volume 131, Issue 4, 28 July 2009.
The cover features an image from the article "Relative abundance and structure of chaotic behavior: The nonpolynomial Belousov–Zhabotinsky reaction kinetics" by Joana G. Freire, Richard J. Field, and Jason A. C. Gallas.
Abstract:
We report a detailed numerical investigation of the relative abundance of periodic and chaotic oscillations in phase diagrams for the Belousov–Zhabotinsky (BZ) reaction as described by a nonpolynomial, autonomous, three-variable model suggested by Györgyi and Field [Nature (London) 355, 808 (1992)]. The model contains 14 parameters that may be tuned to produce rich dynamical scenarios. By computing the Lyapunov spectra, we find the structuring of periodic and chaotic phases of the BZ reaction to display unusual global patterns, very distinct from those recently found for gas and semiconductor lasers, for electric circuits, and for a few other familiar nonlinear oscillators. The unusual patterns found for the BZ reaction are surprisingly robust and independent of the parameter explored.
This is the cover page of Soft Matter, Volume 7, Issue 7, 7 April 2011.
The cover features an image from the article "Shape- and size-dependent patterns in self-oscillating polymer gels" on page 3141 by Irene Chou Chen, Olga Kuksenok, Victor V. Yashin, Ryan M. Moslin, Anna C. Balazs, and Krystyn J. Van Vliet.
Abstract:
Despite many advances in designing biocompatible materials, inflammation remains a problem in medical devices and implants. We report two methods, microcontact printing and photodegradation by UV exposure, to pattern dextran and hyaluronic acidon glass, as well as demonstrate their utility for use as an anti-inflammatory biomaterial. The dextran/glass patterned surface can be further modified by grafting hyaluronic acidto glass, creating a binary polysaccharide patterned surface. We used two geometries, 90 μm squares and 22 μm stripes, to study the human macrophage (THP-1) adhesion on the patterned surfaces containing dextran, hyaluronic acid and the binary pattern. The results indicate that a majority of the macrophages are non-adherent on hyaluronic acidfor three day culture. The ranking of surfaces according to macrophage adhesion is 3-aminopropyltriethoxysilane-modified glass culture dish, dextranized surfaces, glass, and hyaluronic acid-modified surfaces. On the binary pattern of dextran and hyaluronic acid, macrophages preferentially attach and adhere to the dextranized area. Patterned surfaces provide an excellent platform for mimicking the complexity of the glycocalyx and investigating the interface between this surface and cells. This binary polysaccharidepattern also offers a new route to address anti-inflammatory potential of surface coatings on biomaterials in a high through-put fashion.
This is the cover page of Chemical Communications Volume 50, Issue 74, 25 September 2014.
The cover features an image from the article "Coupled chemical oscillators and emergent system properties" on page 10758 by Irving Epstein.
Abstract:
We review recent work on a variety of systems, from the nanometre to the centimetre scale, including microemulsions, microfluidic droplet arrays, gels and flow reactors, in which chemical oscillators interact to generate novel spatiotemporal patterns and/or mechanical motion.
This is the cover image of Physical Review Letters, Volume 119, Issue 5, 4 August 2017.
The cover features an image from the article "Features of Chaotic Transients in Excitable Media Governed by Spiral and Scroll Waves" on page 054101 by Thomas Lilienkamp, Jan Christoph, and Ulrich Parlitz.
Snapshot of spiral waves resulting from the spatiotemporal dynamics of excitable media. White circles indicate the phase singularities or organizing centers of the waves.
Abstract:
In excitable media, chaotic dynamics governed by spiral or scroll waves is often not persistent but transient. Using extensive simulations employing different mathematical models we identify a specific type-II supertransient by an exponential increase of transient lifetimes with the system size in 2D and an investigation of the dynamics (number and lifetime of spiral waves, Kaplan-Yorke dimension). In 3D, simulations exhibit an increase of transient lifetimes and filament lengths only above a critical thickness. Finally, potential implications for understanding cardiac arrhythmias are discussed.
This is the cover image of The Journal of Physical Chemistry C, Vol.122, Number 24, 21 June 2018.
The cover features images of the article "Chemical Wave Patterns and Oxide Redistribution during Methanol Oxidation on a V-Oxide Promoted Rh(110) Surface" on page 12694 by Bernhard von Boehn and Ronald Imbihl.
Abstract:
Chemical wave patterns and the formation of macroscopic vanadium oxide islands have been investigated in the 10–4 mbar range during catalytic methanol oxidation on ultrathin VOx films (θV ≤ 1 monolayer equivalent) supported on Rh(110). At temperatures around 800 K, wave fragments traveling along the [11̅0] direction and oxidation/reduction fronts exhibiting different front geometries are observed with photoemission electron microscopy. At ≈1000 K, a redistribution of VOx leads to the growth of macroscopic oxide islands under reaction conditions. On these macroscopic V-oxide islands chemical waves including traveling wave fragments propagate. Under conditions close to equistability of oxidized and reduced phase, a dendritic growth of the V-oxide islands is observed. In contrast to Rh(111)/VOx, almost no catalytic activity in formaldehyde production is found on Rh(110)/VOx.
This is the cover image of «КВАНТИК» №6, 2018
The cover features an image from the article "Борис Белоусов: О первых исследователях химической реакции, которая всё время идёт по кругу (Boris Belousov: About the first researchers of a chemical reaction that goes on in a circle all the time)" by Marina Malchonov.