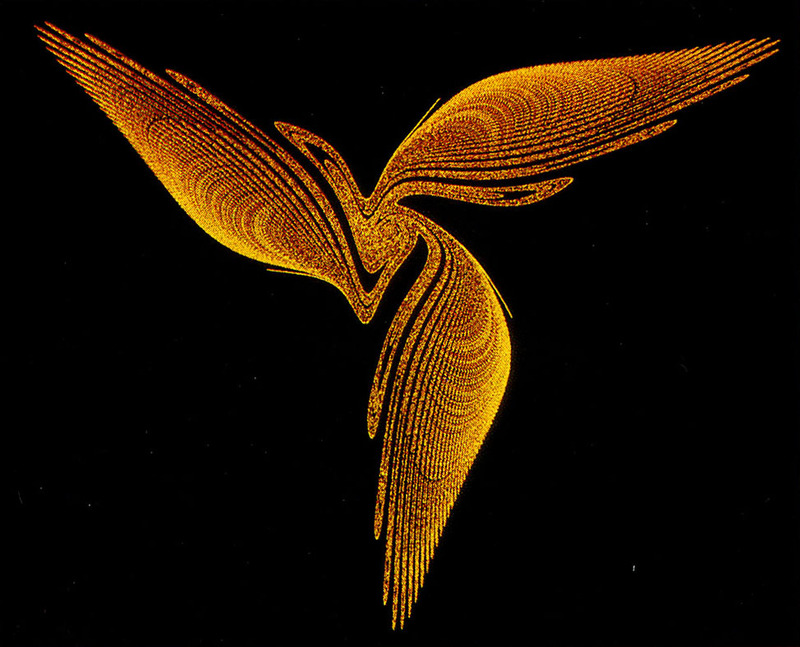
M1 - 'Three-winged' pattern of a chaotic attractor.

M1 - 'Three-winged' pattern of a chaotic attractor.

This image portrays the chaotic attractor according to a formula by I. Gumowski and C. Mira, which consists of quotients of polynomials. The variation of parameters leads to a manifold of patterns like the “three-winged” in this image. The repeated application of the formula renders points which move around unpredictably. Only large numbers of such points form organized structures, as shown here.

Pattern formation (Physical sciences); Physics; Visualization; Mathematics; Mathematics in art; Lyapunov exponents;Nonlinear Dynamics; Pattern Formation; Chemical Waves; Reaction Diffusion Systems; Nonlinear Waves; Traveling Waves; Chemical Oscillations; Oscillating chemical reactions;

Markus, Mario

Dynamic Pattern Formation in Chemistry and Mathematics: Aesthetics in the Sciences: Catalogue of an Exhibition

Müller, Stefan C; Plesser, Theo; Max-Planck-Institut für Ernährungsphysiologie Dortmund, West-Germany;

Markus, Mario;

1988

DynamicPattern_P_002

Laupenmühlen Druck, Bochum

JPEG

Still Image